The relation
of total cost to volume of operations has its most important application in
management accounting, but is also used in cost finding. It is important in
management accounting because managers frequently face decisions involving
changes in volume of operations (along with other changes). To determine the
profit impact of a decision, it is necessary to predict the resulting changes
in cost. This requires knowledge of existing cost-volume relations. In cost
finding, a predetermined overhead rate (burden rate) can be calculated only
after the total amount of overhead cost for the year has been predicted. Since
the volume of output is important to the amount of variable costs which should
be expected, it is necessary to know the cost-volume relation in order to
predict the total amount of overhead cost.
The relation
of cost to volume generally falls into two categories, fixed and variable,
explained below. Other possibilities exist, and the most important
one—semivariable costs—will also be discussed.
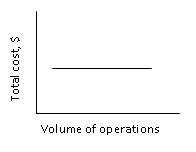
Fixed Costs. A fixed cost is one for which the total
amount of the cost per period is independent of the volume of operations,
within a relevant range of volume. Graphically, it can be shown as indicated in
Fig. A-1. The graph shows that as the volume of operations fluctuates within
the relevant range, as shown
FIG. A-l Graph of a fixed cost.
by the length of the line, the total
amount of this expense, as measured on the vertical scale, remains constant.
Examples include the salary of the factory manager, property taxes, and
insurance on the factory building and equipment. Note that the definition does
not say that a fixed cost will never change. Managers know that salaries,
taxes, and insurance do change. The significant point is that the amount of the
fixed cost is not directly changed by changes in volume. It would be most
unusual, for example, if a factory manager's salary were to fluctuate from
month to month based on the production volume of the factory. (If this were to
happen, the salary would no longer be an example of a fixed expense.) If there
is u significant
expansion of the factory capacity, the factory manager's salary might be
increased at the next salary review. In addition, the expansion of factory
capacity would probably increase the amount of insurance and taxes. However,
these changes would not make these costs variable costs. Rather, the amount of
cost would have changed from one fixed level to another fixed level. The new
line on the graph would be higher than the old line, but it would still be
horizontal. If this were to happen, manage ment would have to replan a variety
of activities anc^ also alter the overhead rate used in the factory.
Variable Costs. A variable cost is one in which the
total amount varies in direct proportion to tht, volume of operations
but the per-unit cost remain constant within a relevant range. This can be illustrated
as shown on the graph in Fig. A-2. To meet this
FIG. A-2 Graph
of a variable cost.
rather strict definition, the line of the
variable cost must be pointed so that it would pass through the origin of the
graph (0,0), if the relevant range extended that far back. A prime example of a
variable cost is direct materials used in the production of a product.
Increasing production by 10 percent will increase the amount of direct
materials used by 10 percent. Further, one should expect that a reduction in
volume of operations by, say, 15 percent, would reduce the amount of direct
materials required by 15 percent. This is because the amount of direct
materials used per unit of product is constant.
The idea of
a relevant range is important because experience shows that if a manager were
to consider doubling volume or cutting volume by two-thirds, cost levels would
change in an erratic manner. But such large changes are the exceptions; dealing
with them requires a special study. In the normal situation, managers have
found that cost can be expected to fluctuate in a predictable manner within the
relevant range in which most decisions are made.
Semivariable Costs. If a cost increases as a result of
volume changes, it cannot, by definition, be a fixed cost. But there are costs
which increase as a result of volume changes but do not fit the rather strict
definition of variable cost. Maintenance and electricity costs are examples.
These costs often fall into the category of semivariable costs. Within the
relevant range, a semivariable cost will increase as a result of changes in the
volume of operations but not in direct proportion to volume. A graph of a
semivariable cost is shown in Fig. A-3. Note that the line
FIG. A-3 Graph of a
semivariable cost.
slopes upward as volume increases but that
it would not pass through the origin if the relevant range extended back that
far.
Semivariable
costs present no new problems in analysis, however, because they can be broken
into a fixed component and a variable component. This can probably be seen most
easily by referring to the graph in Fig. A-4. The graph is the same as that in
Fig. A-3
FIG. A-4 Graph of a semivariable cost showing
fixed and variable components
except that the dashed line is added to
illustrate that the semivariable cost can be thought of as a variable cost with
a fixed amount added on top. The dashed line shows the variable-cost component.
The amount added on top is a fixed amount, the same at all volumes, thus
fitting the definition of a fixed cost. For analysis, a semivariable expense is
broken into its fixed and variable components.
Considering
the examples of maintenance and electricity, one can understand why a fixed and
variable component would exist. The routine preventive maintenance is the
fixed component. The balance of the maintenance could be expected to increase
or decrease as the volume of operations resulted in greater or less use of the
machines. Electricity used ini lighting is likely to be a fixed cost. The
plant must be- lighted whether it operates at 70 percent capacity or 80 percent
capacity; the lighting cost does not vary with volume. The electricity used to
power the machines, however, could be expected to increase or decrease as the
volume of operations resulted in greater or less use of the machines. Thus, the
total elewrmcity cnat would have a fixed and a variable component; therefore it
would be a semivariable cost.